Goal: test of CPT symmetry using antihydrogen
Fundamental symmetries and resulting conservation laws are a fundamental concept in physics. In particle physics, it was believed that nature conserves the symmetries of space until the discovery of parity (P) and later charge conjugation and parity (CP) violation. One of the cornerstones of the standard model (SM) of particle physics is the combined application of charge conjugation, parity, and time reversal: CPT. The proof that this symmetry is conserved in the SM originates from the properties of the quantum field theories used and is based on a mathematical theorem. Whether the conditions generating this mathematical theorem are valid in nature is an important question to answer experimentally.
Further interest arises in studies of CPT symmetry from the fact that certain prerequisites of the mathematical proof like point-like particles are not any more valid in string theory, so that an observation of CPT-violation could be a first hint for the validity of string theory and a clear evidence for physics beyond the standard model.
A hint that matter-antimatter symmetry is violated comes from the observed absence of antimatter in the universe. This can be explained by the famous Sakharov criteria that include C and CP violation. The experimentally observed CP violation in the K and B meson sector, however, is quantitatively not strong enough to explain the large antimatter asymmetry in the universe. An even tiny violation of CPT symmetry during the Big Bang could have led to a creation of more matter than antimatter, which remained after annihilation, thus creating the observed matter dominance.
This experiment will be the first precise hyperfine spectroscopy experiment of an atom consisting purely of antimatter. The ground-state hyperfine structure offers one of the most sensitive tests of CPT symmetry, whose investigation is of extreme importance in searching for new physics beyond the current understanding of particle physics and a possible source of the antimatter absence in the universe.
Advantages of antihydrogen for testing CPT
Antihydrogen, a bound state of an antiproton and a positron, is the simplest stable atom consisting entirely of antimatter. Since its hydrogen counterpart is one of the most precisely studied systems in physics, a comparison of antihydrogen and hydrogen offers one of the most sensitive tests of CPT symmetry. The two most precisely measured transitions in hydrogen are the optical 1S-2S two-photon transition and the microwave ground-state hyperfine (HFS) transition at 1.4 GHz, which are known with relative precision of 10–14 and 10-12, respectively. By measuring them with similar precision for antihydrogen, a very sensitive test of CPT symmetry can be performed.
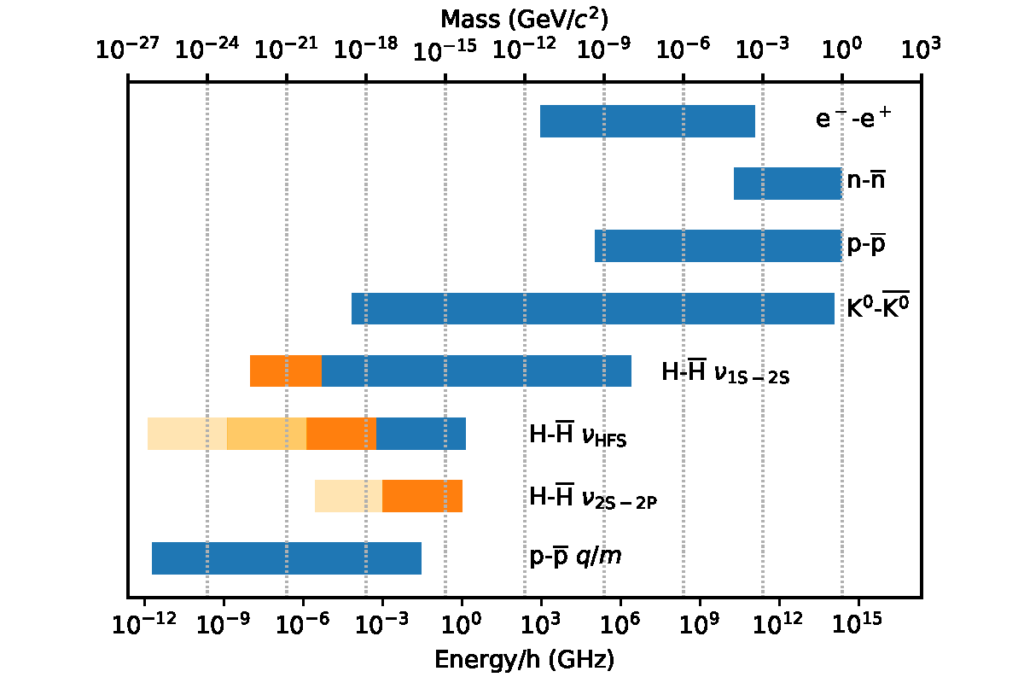
Furthermore, based on the argument that any CPT-violating interaction must manifest itself by a term in the Dirac equation that has the dimension of mass or energy, the highest sensitivity can be obtained by reaching the highest precision in terms of absolute energy. This argument has been brought forward by Kostelecky et al. in their extension of the standard model SME [1,2], which is the only model that allows a comparison of CPT tests in different systems. Often the mass difference between the neutral kaon and antikaon is referred to as the best test of CPT since it is known with a relative precision of 10–18. On an absolute mass scale, this value corresponds to a frequency of 100 kHz, which is much larger than what can be achieved in high-precision spectroscopic measurement of atomic transitions. Among them, the antihydrogen HFS transition could potentially obtain one of the best values on an absolute scale (see Fig. 1).
Like CP violation, which is observed so far only in K and B meson decays, CPT violation may also appear only in a certain area of particle physics. Therefore measurements involving all types of particles and interactions (in Fig. 1 results are listed for leptons, baryons, meson, and atoms) are needed.
The study of the HBAR-HFS is complementary to the 1S-2S laser spectroscopy. While the latter is dominated by the electromagnetic interaction and the energy levels are in first order sensitive to the well-known positron mass (which dominates the reduced mass), the HFS is caused by the spin-spin interaction. From a relative precision of ~10–5, correction terms arising from the finite antiproton charge and magnetic radius play a role and the comparison between hydrogen and antihydrogen is also sensitive on possible strong-interaction induced differences in the internal structure of proton and antiproton.